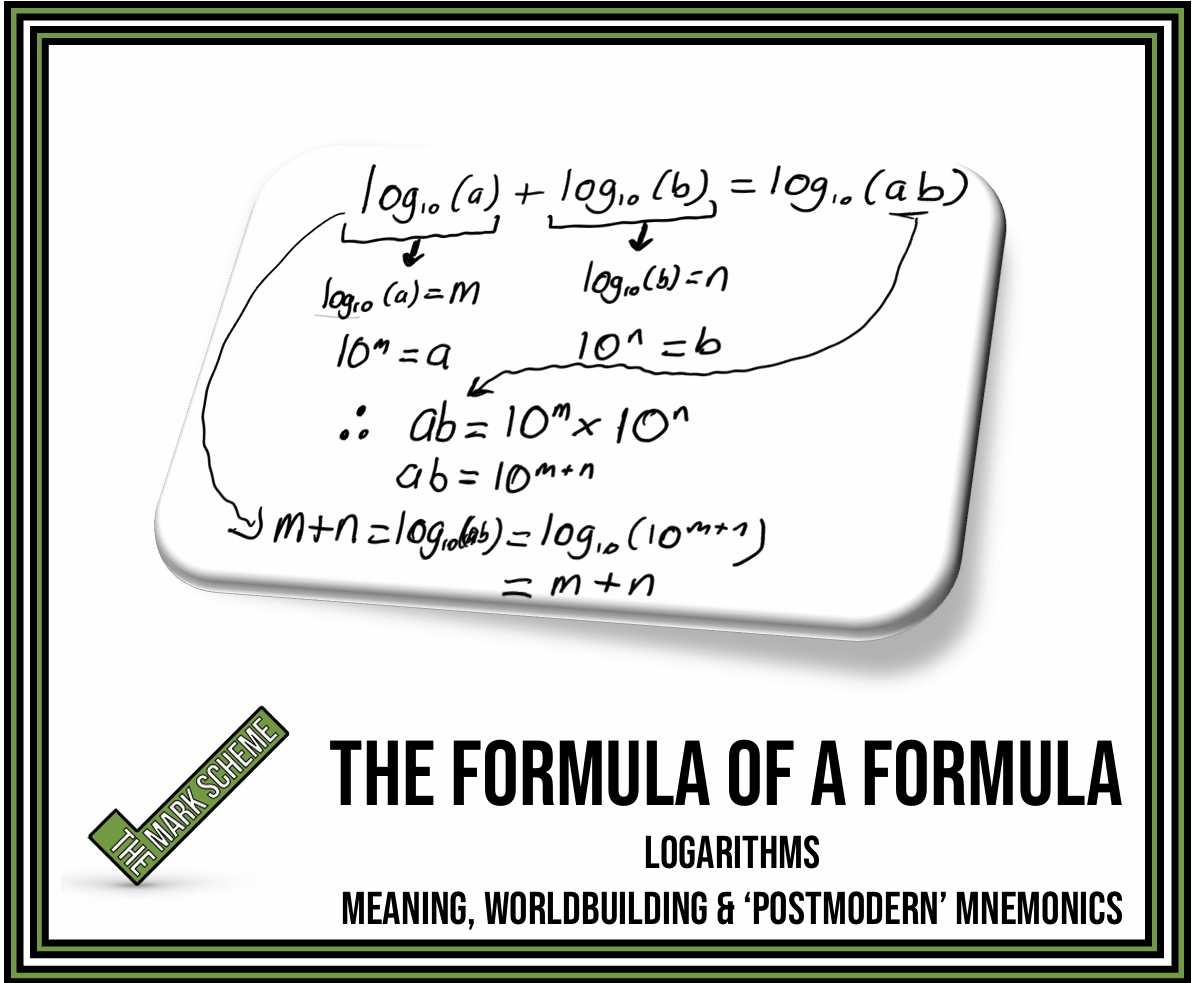
“I can only do it when I understand it”. As maths teachers we hear this one a lot, generally from a student wanting to criticise a prior learning experience they have had. On the surface it is a logical statement, until you reflect that to some extent, we are all unreliable narrators, and young people, who are still learning their own mind, and as said are often looking to ‘pin blame’ on a certain place, or to explain a performance level they were not happy with, can be particularly unreliable.
In the first lesson with a year 12 cohort, I will ask the very ‘basic’ question – “Give me the definition of the mean”. I have done this for more years than I can remember at this point. I have had dozens of the most confident answers – which I then turn back around and say, “That’s a calculation, not a definition”. Then a moment of realisation happens, and we go into further discussion.
One day I will have someone give a good definition of the mean, I am sure, but as of yet, it is yet to happen.
So why do this? Well, it serves a few purposes;
- It allows the students to realise early on that even ‘basic’ mathematical concepts they ‘know’ – there is still new knowledge and skills to develop.
- It allows a reflection point for comments of “I can only do it when I understand it” and its ilk.
- It allows for the ability to reframe and articulate what ‘meaning’ is.
I’ve yet to have a student in a year 12 class who cannot ‘do’ the mean. But I have yet to have a student articulate a clear and comprehensive ‘definition’. So, do they really “understand it”?
What does this have to do with logarithms?
Fair question. Well logarithms are a notoriously challenging aspect of the A Level Mathematics specification – particularly within year 1. Before even getting into application there are a lot of rules and facts that students need to remember in order to begin to access the question, and any misremembering of the rules will come with high penalties. There have been a number of different studies globally relating to the misconceptions and errors that learners have with regards logarithms and their applications. Whilst a number of these will be touched upon in this post, I will be focussing more on broader teaching strategies that I employ in the classroom to deal with this topic, continuing form the introduction post, relating to the “Laws of the land, the laws of your classroom”.
Worldbuilding – “Build & Destroy” – KEY 4
Great teaching requires the explicit development of routine. Routine is often encompassed with behaviour management strategies, but ideally it goes beyond that – a lot of emphasis currently is on metacognition, but it is still important to develop and introduce the key cognitive processes you want students to undertake or get used to within class. One of the earliest aspects I emphasise within mathematics is ‘build & destroy’. This is a rather sensationalist way of discussing inverse processes – but it is one to resonate and become memorable to learners. In maths we learn to ‘build’, we then learn to ‘undo’/’destroy’. Addition and subtraction, multiplication and division, squaring and square-rooting. They are concepts that students are familiar with and can easily recognise as inverse processes.
Within AS Maths there are a category of topics that I refer to as the “Key 4” of Pure Mathematics. These are differentiation, integration, trigonometric equations and identities, and exponentials and logarithms. This is to emphasise these four topics importance to higher level study, but also due to their extension far beyond GCSE knowledge students will have entered the programme with. These key 4 topics all have a link to this idea of ‘build’ & ‘destroy’. Differentiation and indefinite integration are inverse processes – and are introduced as such by most textbooks and teachers. You can use these topics to continue to emphasise this aspect of inverse processes, which can then be built upon when students reach trigonometry.
A bit like the mean example, students, very rarely have a strong understanding of sine/cosine/tan – or at least one that stands up for scrutiny. They instead pin knowledge to the ‘formulae’ or SOHCAHTOA. What they ‘are’ is unimportant to their ability to ‘do calculations’. Similarly, sin-1/arcsin are just buttons on a calculator that allows them to find an angle. However, trigonometry is a fantastic link into logarithms. Given students prior introduction to trigonometric ratios they serve as the next step of working with inverse processes. Researchers such as Kenney and Kastberg (2013) highlight this as well saying of the link between exponentials and logarithms, “It is also similar to their interaction with other transcendental functions such as f(x) = sin(x)”.
I will generally group the two topics of trigonometric equations and identities, and exponentials and logarithms together in my curriculum plan. As whilst they are dramatically different skills, there is an underpinning of inverse functions which can be vital for developing a working knowledge base for logarithms.
“I don’t understand logarithms?” – What is a name?
“Someone decided you were important enough to give a specific noise too, in order to identify you. That is all a name is.”
One of the most immediate barriers I have found to logarithms is that students get hung up on the word. When they say the don’t understand logarithms, it’s not actually the mathematical content, they don’t understand the word and that acts as a cognitive block for any other aspects you want to build on top of.
Now this block doesn’t exist for trigonometry in my experience, as students will pin their ‘knowledge’ to a formula instead. It doesn’t matter why sine is called sine, or why tangent is tangent – they just are, and sine is opposite/hypotenuse. But ‘logarithm’ causes issues. Thompson and Rubenstein (2000) indicate the vocabulary are the ‘surface structures’ that are used to transmit ideas to students in discussion that will lead to the development of the ‘deep structures’ of mathematical concepts. If learners can’t access the vocabulary, how can they access the mathematical concepts?
So again, you use trigonometry as a primer topic, build the behaviours on something they are already familiar with. Yes you can discuss etymology, but as pointed out by Weber (2016) “The meaning of the term “logarithm” is not self-evident; and nor is the meaning of its etymological translation: “ratio number.”…” – but ultimately, it’s something important enough to be given a ‘noise’.
A function and a number? – The ‘Postmodern’ Mnemonic
Beyond terminology, one of the issues with logarithms are that they are two different things in one. They are both a mathematical function – the inverse function of an exponential, but also a representation of a number. The syllabus puts a greater emphasis on the function aspect of things, however logarithms existed as a process before the algebraic definition of an exponential function was created (Panagiotou, 2011). Logarithms were contextualised more in-line with division historically, and notation such as 8//2=3 were used instead of log2(8)=3. Indicating that 8 divided by 2, three times, gives an answer of 1 (Weber, 2016). Logarithms interpreted more as a format of division as opposed the inverse of an exponential function. Again this can provide a reference point to calculus, as similar to differentiation/integration – as part of mathematical syllabus differentiation and exponentials come first, however logarithms and integration were the first discovered mathematical concepts.
Implicit references to historical methods are often used within the topic already, such as the work of Bernoulli and compound interest in order to define ‘e’, but it is rare to see this with logarithms themselves. The writing of Panagiotou (2011) includes a number of interesting ways that logarithms could be explored from a historical context, that I will be looking to adapt for future teaching. There is also an interesting broader point raised regarding mathematical curricula – about how textbooks and specifications focus on the final ‘conceptual facts’ and not the efforts and failures that lead to the concepts – arguably which is what mathematics truly is.
Rafi and Retnawati (2018) indicate that students often see “log” as a variable, reconfirming findings of Liang and Wood (2005) where “students often see the notation for logarithm “log” as an object rather than as an operation”. This leads to situations such as log(2x+8)=4 becoming log2x+log8=4, students incorrectly believing the bracket can be expanded. Empirically this is often exacerbated by the instructions of teachers to treat logs ‘like algebra’ when discussing collecting like terms – this can be misinterpreted or misapplied by the learner leading to situations like this. As mentioned earlier, this problem is conceptually similar to problems faced in trigonometry, such as sin(4x+20)=0.5. Developing skills and knowledge in one area can then be used to strengthen initial understanding of logarithms during their introduction, and avoid issues with wrong intuition on behalf of the student, a concern identified in the work of Dimtarini (2018).
As well as misapplication and overgeneralisation of mathematical rules in processes, research often finds that learners do not appreciate logarithms as numbers. Berezovzski and Zazskis (2006) show that even students who are adapt at solving logarithmic problems don’t recognise logarithms as numbers. In research from Liang and Wood (2005) only 6% of the student respondents were able to evaluate the expression 2log2(5). This is interesting, as whilst emphasis of delivery is on logarithms defined as inverse functions of exponentials, it shows that a true appreciation of that concept is not engrained for students to apply to this problem. However, this problem would also be much more accessible with an understanding that a log2(5) is just a number. Verbalising the statement as “2 to the power of, the number where if 2 is raised to the power of it, the answer is 5”.
During my teacher training I had a lecturer who used a ‘Postmodern Mnemonic’ to support understanding of logarithms. He broke the notation of LOG down to “L for for ‘What’, O for ‘Power’ and G for ‘Of’…” – it was ridiculous, it was played off as ridiculous, but it worked, and it works. It emphasis that a logarithm is a number, and supports in the ability to convert between logarithms and exponentials, without having to just rote learn a formula. It is a very simple but affective method to support in the understanding of logarithms, as it is both a memory device, but also literally verbalising of the numerical meaning simultaneously, and is a great launchpad for developing understanding as to why you cannot find the logarithm of a negative number.

Linking to Prior Knowledge
A large portion of this is looking at how to activate prior knowledge to provide meaning to students, and pin new knowledge to existing schema. Developing an appreciation for “build and destroy”/inverse mathematical processes, for simple processes, but also calculus, trigonometry and then exponentials and logarithms.
There are linguistic strategies that accompany this, emphasising the link between the ‘base’ of a logarithm and the ‘base’ of the exponential is a key way of emphasising the inverse relationship between the functions consistently in the classroom, and then the ‘postmodern mnemonic’ can to develop appreciation of the numerical aspect of a logarithm, providing learners with a way of verbalising and articulating the affect of a logarithm on the input – a challenge the notation provides (Hurwitz, 1999 via Kenney and Kastberg, 2013)
This all needs to be coupled with other strategies, these are entry barriers to the topic I am addressing. Again, it is vital to link back to prior knowledge. The link between exponentials and logarithms is a key aspect, but linking the multiplication/division log laws to the basic laws of indices, gives another opportunity to emphasise the ‘inverse’ link.

There are also opportunities to illustrate correct mathematical procedure by tying back to common methods such as Pythagoras’ Theorem.

Logarithms and exponentials are always going to be a challenge for students. But these are strategies employed within my classroom to support with developing initial understanding and allow students to begin to develop meaning of mathematics.
With all things, based on areas of emphasis when constructing curriculum there is going to be a trade-off. For me currently, it is natural logarithm – whilst observationally, standard base logarithms are handled with a higher quality, the transition to including natural logarithms has been less smooth. But like all things in the classroom, as long as you are aware, you can prepare to tackle the shortcomings.
References and Reading:
Berezovski, T. and Zazskis, R. (2006). Logarithms: Snapshots from two tasks, Proceedings of the 30th Conference of the International Group for the Psychology of Mathematics Education, vol.2, Pg.145-152
Dintarini, M, (2018). Understanding Logarithm: What are the Difficulties That Students Have?. Advances in Social Science, Education and Humanities Research, vol.231
Kenney, R. and Kastberg, S. (2013). Links in learning logarithms. Australian Senior Mathematics Journal, vol.27(1)
Liang, C.B. and Wood, E. (2005). Working with Logarithms: Students’ Misconceptions and Errors. The Mathematics Educator, vol.8(2), Pg.53-70
Panagiotou, E.N. (2011). Using history to teach mathematics: The case of logarithms. Sci & Educ, vol.20 , Pg.1–35
Rafi, I. and Retnawati, H. (2018). What are the common errors made by students in solving logarithm problems?, Journal of Physics: Conference Series
Thompson, D.R. and Rubenstein, R.N. (2000). Learning Mathematics Vocabulary: Potential Pitfalls and Instructional Strategies. The National Council of Teachers of Mathematics.
Weber, C. (2016). Making Logarithms Accessible – Operational and Structural Basic Models for Logarithms. Journal fur Mathematik-Didaktik, Vol.37 (1), Pg.69-98